Provide by:- bca-wala.blogspot.com
Ankit Rajbansi😍
Unit-5:-Vector alegbra
Introduction to Vectors

DEFINITION: Vectors were developed to provide a compact way of dealing with multidimensional situations without writing every bit of information. Vectors are quantities that have magnitude and direction, they can be denoted in three ways: in bold (r), underlined (r) or .
The position point of a vector is defined using Cartesian co-ordinates: it uses the coordinates of the OX, OY and OZ axes where O is the origin. We will be looking at vectors in 3 dimensional space in Cartesian coordinates. Similar ideas hold for vectors in n dimensional space (n-vectors).

Addition and Subtraction of vectors
DEFINITION: We will look at two laws involving addition and subtraction; The commutative law and the Associative law.
The commutative law:
Addition and subtraction of vectors obeys the commutative law,


This means that:

In terms of the following components

For addition:

For subtraction:

The Associative law:
This uses different routes to get to the same final desination


In terms of the following components:


The length and unit vector of a vector
The length of a vector :
DEFINITION: For any vector like this one

The length of a (which is denoted by |a|) is given by:

The unit vector of a vector
DEFINITION: A unit vector is a vector with unit length 1. By standard convention we let i, j and k be unit vectors along the positive x, y and z axes, so in terms of components:


Scalar multiplication of vectors
DEFINITION: This involves the multiplying of a vector by a scalar, i.e. a number.
In terms of the following components:


Scalar multiplication will change the length of the vector and if the factor λ is negative the vector will point to the opposite direction. Scalar multiplication satisfies the following properties where a and b are vectors, λ and μ and are scalars.

Scalar product
Let us take two vectors.

Then the scalar product of a and b, denoted by a.b is

Setting these equal to each other gives

The angle between two vectors
Using the two above formulae and setting them equal to each other as shown below we are able to calculate the angle between two vectors.

Vector Product
DEFINITION: The vector product is fundamentally different from the scalar product. The vector product of two vectors is a vector but the scalar product is a scalar. The vector product is given by:

where
|a| is the length of a
θ is the angle between vectors
n is the unit vector perpendicular to a and b whose direction is determined by the left hand skew rule.

For vectors a × b is found by using the following:

For simplicity this can be written in terms of determinants.

Scalar Triple Product
By the name itself, it is evident that scalar triple product of vectors means the product of three vectors. It means taking the dot product of one of the vectors with the cross product of the remaining two. It is denoted as
[a b c ] = ( a × b) . c
The following conclusions can be drawn, by looking into the above formula:
i) The resultant is always a scalar quantity.
ii) Cross product of the vectors is calculated first followed by the dot product which gives the scalar triple product.
iii) Talking about the physical significance of scalar triple product formula it represents the volume of the parallelepiped whose three co-terminous edges represent the three vectors a,b and c. The following figure will make this point more clear.
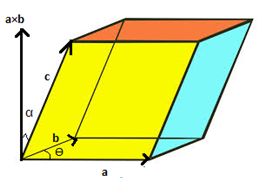
According to this figure, the three vectors are represented by the coterminous edges as shown. The cross product of vectors a and b gives the area of the base and also the direction of the cross product of vectors is perpendicular to both the vectors.As volume is the product of area and height, the height in this case is given by the component of vector c along the direction of cross product of a and b . The component is given by c cos α .
Thus, we can conclude that for a Parallelepiped, if the coterminous edges are denoted by three vectors and a,b and c then,
Volume of parallelepiped = ( a × b) c cos α = ( a × b) . c
Where α is the angle between ( a × b) and.c
We are familiar with the expansion of cross product of vectors. Keeping that in mind, if it is given that a = a1i^+a2j^+a3k^, b = b1i^+b2j^+b3k^ , and c = c1i^+c2j^+c3k^ then,we can express the above equation as,



Try to recall the properties of determinants since the concept of determinant helps in solving these types of problems easily.
iii) If the triple product of vectors is zero, then it can be inferred that the vectors are coplanar in nature.
The triple product indicates the volume of a parallelepiped. If it is zero, then such a case could only arise when any one of the three vectors is of zero magnitude. The direction of the cross product of a and b is perpendicular to the plane which contains a and b. The dot product of the resultant with c will only be zero if the vector c also lies in the same plane. This is because the angle between the resultant and C will be 90∘ and cos 90∘..
Thus, by the use of the scalar triple product, we can easily find out the volume of a given parallelepiped




